A temperature-composition diagram is shown for two liquids (A, B) that are only partially miscible within the region enclosed by the orange and pink curve. Each phase in the two-phase region contains both A and B; the \(\alpha\) phase (represented by the pink line, mole fraction \(x_A^{\alpha}\)) is enriched in A and the \(\beta\) phase (orange line, \(x_A^{\beta}\)) is enriched in B. Outside the phase envelope, A and B are completely miscible. Sliders for temperature and overall mole fraction of A move the black dot around the diagram. The sizes of the rectangles at the top for pure A and pure B are proportional to the overall mole fraction of that component. The sizes of the containers on the right are proportional to the amounts of the phases (either \(\alpha\) and \(\beta\) or a single miscible phase) in equilibrium, and the mole fractions are represented by the relative numbers of green (A) and blue (B) circles.

The temperature-mole fraction diagram shows phase separation for a binary liquid mixture of component A (green) and component B (blue). The region under the orange and pink curves corresponds to two liquid phases in equilibrium. For a composition in the two-phase region, a horizontal line intersects the orange and pink curves at the compositions of the two phases that are in equilibrium. The lever rule is used to determine the amounts of \(\alpha\) and \(\beta\) phases. $$\frac{x^\alpha_A -x_A}{x_A-x_A^\beta} = \frac{n^\beta}{n^\alpha}= \frac{\text{number of moles of β phase}}{\text{number of moles of α phase}}$$ where \(x_A\) is the overall mole fraction of A.

That is, the amount of \(\beta\) phase is proportional to the length of the horizontal dashed orange line, and the amount of the \(\alpha\) phase is proportional to the length of the horizontal dashed pink line.

This simulation was created in the Department of Chemical and Biological Engineering, at University of Colorado Boulder for LearnChemE.com by Rachael Baumann under the direction of Professor John L. Falconer and was converted to HTML5 by Drew Smith. This simulation was prepared with financial support from the National Science Foundation. Address any questions or comments to learncheme@gmail.com. All of our simulations are open source, and are available on our LearnChemE Github repository.

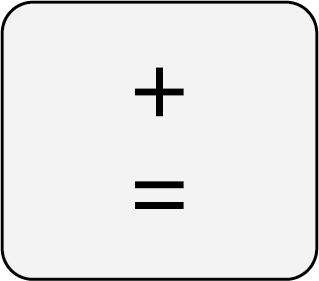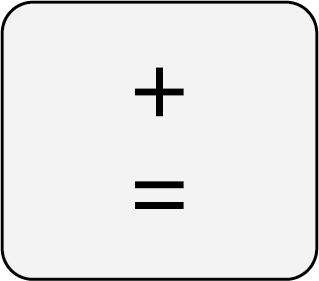If this simulation is too big for your screen, zoom out using  +  on Mac or  +  on Windows. To zoom in, use  +  on Mac or  +  on Windows.